If I had a penny for every question about how to differentiate multiplication… Well, I bet you can guess!
Multiplication is tricky to construct. Some students notice multiplication patterns quickly. They are eager for more! But, many students also struggle.
That is why ways to differentiate multiplication instruction is always on my mind when starting our multiplication unit. I aim to have open-ended, low floor, and high ceiling tasks that meet students at their working level.
I’ve shared some of my favorite ways to teach multiplication, including:
- Hands-On Ways to Teach Multiplication
- Favorite Manipulatives to Teach Multiplication
- How to Build Multiplication Fact Fluency
- Favorite Multiplication Centers
- How to Introduce Multiplication
With all of that in mind, today, I have compiled my most successful ideas for how to differentiate multiplication instruction and practice in the classroom. The majority of these tips are open-ended activities that allow students to work at their exact working number and level of understanding.
Differentiate Multiplication with Manipulatives
One of the most effective ways to differentiate any lesson is the “take what you need approach.” This approach to differentiating multiplication can take many forms but always includes choice.
- Choice over assignment scaffolding
- Choice over the numbers they use to solve an open-ended problem
- Choice over what supports to use
Enter: Math manipulatives! Manipulatives are the most helpful scaffolding and support for any math assignment. They are particularly useful to differentiate multiplication activities.
And it is simple. A math manipulative becomes available after learning how to use it. I introduce a lot of math manipulatives at the beginning of the year. That means I’m leaving out a variety of math manipulatives to support understanding ASAP.
Math manipulatives are suitable for ALL students. Need a higher ceiling? A bigger challenge? Ask students to show their thinking physically. Need some ideas?
- Build arrays with square tiles.
- Show repeated addition with unifix cubes.
- Demonstrate how a pattern with a variety of
Need more support? Need something concrete? Multiplication math manipulatives can be relied on to help build a foundational understanding.
Differentiate Multiplication with Games
Games are fun and engaging. Good News: They double as great tools to differentiate multiplication practice.
Differentiating multiplication often takes the form of using higher numbers or lower numbers, depending on a student’s working number.
Games provide a range of difficulty without much prep. You simply print the games with different facts. For example, I love using Bump for differentiating multiplication. Students with a higher working number start with higher or complex numbers (3, 7, 9) on their boards. Students working on more foundational multiplication facts (2, 5, 10) start with those numbers on their boards.

Other easy-to-differentiate games include Flip and Compare and Array Capture. A deck with more difficult cards changes the level of difficulty for Flip and Compare.

On the other hand, I differentiate Square Capture in multiple ways. The size of the game boards and/or the numbers on the spinner increases or decreases the working number. Square Capture is also easily differentiated by using high-sided dice instead of a spinner.
Reconsider Timed Multiplication Tests
As I’ve mentioned before, the memory of timed multiplication tests haunts me from my childhood. Processing speed, attention, anxiety, and choice of strategy all factor into how much time students need to complete multiplication activities. That’s why time is crucial when we differentiate multiplication activities. That includes tests.
Let’s pause for a moment. Math fact efficiency should increase confidence, accuracy, and speed. Timed multiplication tests- the anxiety leading up and the impact after -have a measurable impact on student confidence around multiplication. Students quickly associate speed with knowledge. This idea leads students who take their time to feel less confident than those who speed through. Furthermore, timed tests don’t provide a complete picture of a student’s abilities as a math thinker. Instead, they can have long-term harmful effects.
Instead, I focus on other ways to differentiate multiplication assessment and development.
- I work with students in differentiated, small groups through guided math.
- I break down multiplication facts by fact family.
- I use hundred charts to keep track of multiplication facts that we explore.
- I teach properties of numbers as students see patterns emerge in the hundred chart series.
- I reinforce facts out of their fact family through activities and games such as Multiplication Bump and fact searches (like word searches).

Focused Small Group Work
The most powerful way to differentiate any lesson is focused, small group work.
Differentiating multiplication means providing students scaffolding where they are. From there, you build their multiplication understanding and foundation.
That’s where small groups become your golden ticket: You pull some groups to help create a more solid foundational understanding of multiplication. You meet with other small groups to help enrich and extend their understanding. No matter the need, small groups provide the opportunity to reinforce concepts and extend concepts.
What does that include?
- Demonstrations of, and practice with, hands-on multiplication activities
- Enrichment opporunities that require students to apply what they know
- Guidance through math explorations that can be completed or repeated independently with new numbers

Bonus: Often, students needing enrichment also need support with social-emotional aspects of learning, such as building a growth mindset. I have found that logic puzzles and hands-on activities help those students develop a stronger growth mindset. With me as their guide, they feel more comfortable “failing” and trying activities that seem difficult. That willingness to try transfers to logic puzzles and hands-on activities in independent work.
Multiplication Patterns on the Hundred Chart
Another fantastic way to differentiate multiplication? Find patterns!
Students will find the patterns they are ready to find. Then, you can give them a push!
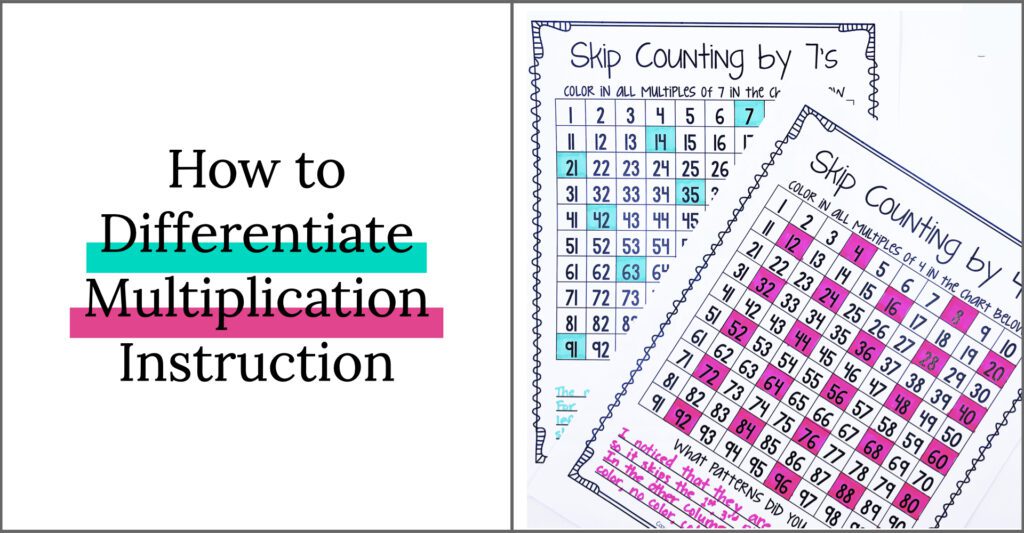
First, I guide students through skip counting exploration. We skip-count on separate sheets of paper, going by multiplication fact families. For example, students use one hundred chart to skip count by 7s. They color in every seven squares, also known as multiples of 7. We do the same thing for 5s on a separate chart.
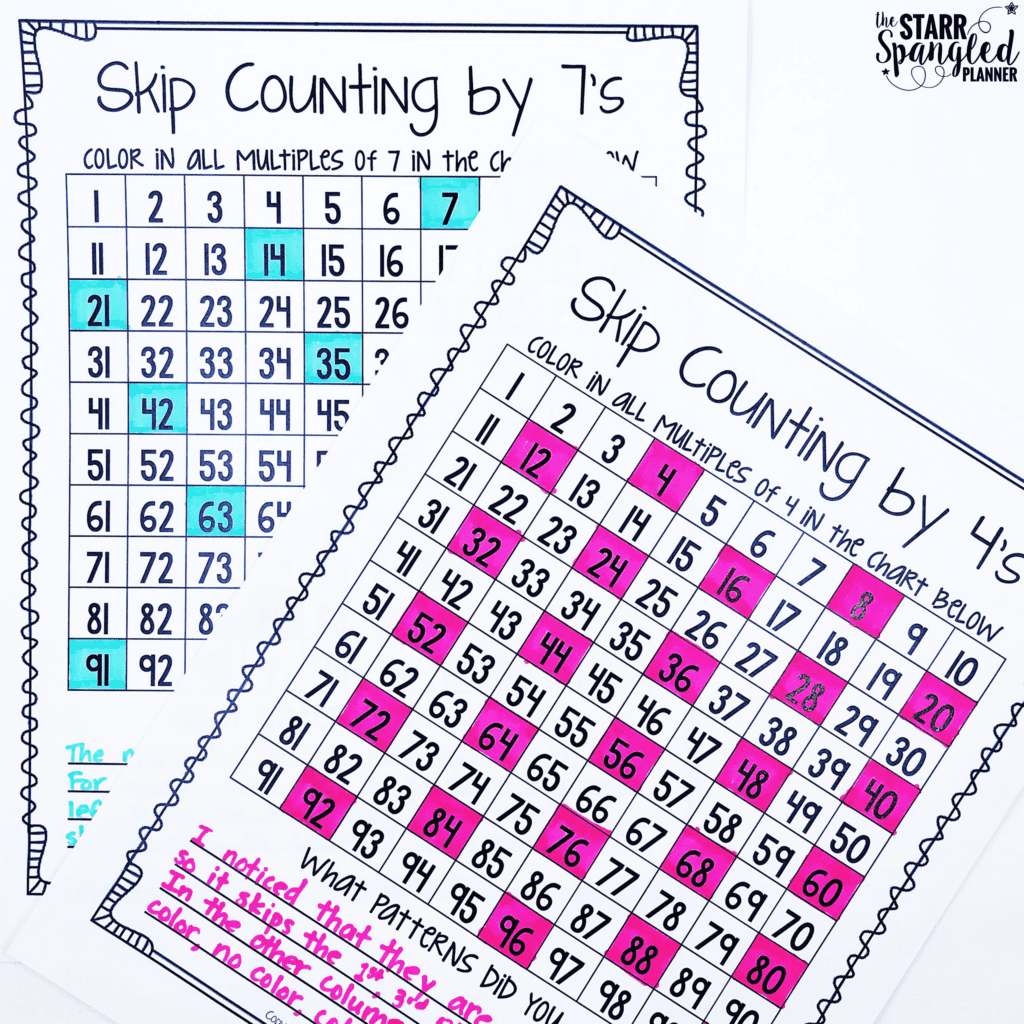
Then, we take it a step further. We use the same hundred chart to color in multiple sets of multiples. For example, 3s and 5s. Each fact family will be in a separate color but on the same chart.
The exclamations of excitement around pattern-finding start quickly! But, a few simple questions that require little prep and practice help students explore more deeply.
- What patterns did you notice?
- Are some numbers colored in more often than others?
- Are some numbers never colored in?
I always ask students to tell me what they noticed. Some students will notice complex patterns. Others will notice the basics. All of their noticed patterns can contribute to meaningful class discussions and further their own understanding of multiplication facts and factors.
Differentiate Multiplication with Explorations
One of my favorite ways to differentiate multiplication is through explorations. Multiplication explorations help EVERYONE construct new understandings of multiplication. However, explorations are a particularly powerful way to extend learning for students who are further along in their understanding.
Those of you who attended my Guided Math Academy have heard me sing the praises of explorations. Explorations, like logic puzzles, help build a growth mindset around math. Why? Because there is no ceiling. Students learn to keep discovering patterns and connections within mathematical concepts.
I typically engage students in multiplication explorations using arrays and factors.
Factors
Every year, I dive deeply into factors through hundred charts. As I mentioned above, a systematic exploration of multiplication facts on a hundred chart is a great way to build student multiplication fact knowledge. Students start to notice patterns, even without me as a guide. They announce phrases such as “They both equal that!” or, “So many numbers land on the 20!” With a few more prompts and structures, students can start to turn those natural noticings into a full-on investigation of the hundred chart.
Arrays
Arrays are an exciting, naturally differentiated exploration in my multiplication unit. We do a variety of hands-on explorations that can scale up or down, depending on the students. Some ideas include:
- Lego creations using arrays
- Array cities
- Simple arrays with square tiles
Scaffolding is critical when introducing arrays. If you scaffold carefully, the rest will follow.
We start by going through numbers 1-36 (or beyond) using square tiles, finding as many arrays as possible for each number. For example, when they get to 12, they should count out 12 tiles. Then list out all of the arrays they can create (1×12, 2×6, 3×4, 4×3, 6×2, and 12×1). Then they can add one tile and see what they can make with 13 tiles.
Again, I guide students using simple questions:
- What do you notice?
- Did you find any patterns when making arrays?
- Can all numbers make arrays? Do they all make the same number of arrays, or can some make more than others?
- What strategies did you use to find all of the arrays for each number?
Arrays are such a powerful visual to support multiplication instruction. I love that it offers so many chances to both support our students who are earlier in their understanding of multiplication, and those who are further along and seeking opportunities to extend their thinking.

Multiplication Mega Pack
Differentiation can be a balancing act. I hope these ideas help you create a multiplication unit that supports all of your students. Have another trick to differentiate multiplication instruction? Let me know in the comments!








Leave a Comment