Mathematical Representations Series Part 5: Contextual Representation

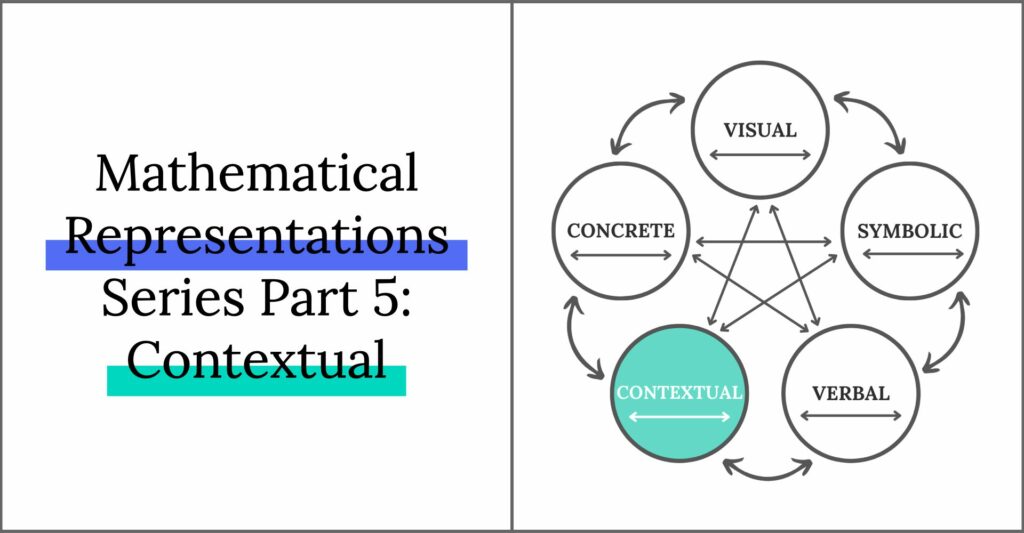
Welcome back to our deep dive into mathematical representations! Today, we are taking a look at contextual representations and how we can translate between symbolic, concrete, visual, and verbal representations. First, let’s do a three-sentence recap of this series so far:

We have already focused on concrete representations and the immense value of manipulatives, and the range of visual representations we want to encourage with our students. We have also looked at how we can use numerals and operations to represent thinking symbolically, as well as how to utilize the power of verbal representations, especially with our pre-literate learners. Our conversation centers around Lesh’s Translation Model, which encompasses the range of ways we represent our thinking, and stresses the importance of making connections between representations.

Today we are talking about contextual representations. Like verbal representations, this is a less discussed representation, because it is not explicitly recognized in the Concrete-Pictorial Abstract model. This is one of the many reasons I like to also consider Lesh’s translation model in addition to the CPA Model (also called CRA).

Contextual Representation

A contextual representation is much like it sounds. We are giving context to an idea. It is how math can be applied in the real world.  Given that we learn math to help us solve real-world problems, it’s a pretty important representation

Example

Let’s look back at the red and yellow counters from our previous discussions. The student had five yellow counters and four red counters. They sketched their concrete representation to create a visual representation. Then they used numerals and symbols, showing 4+5=9 to form a symbolic representation. After this, they verbally shared their understanding and then consolidated that language into a written form.

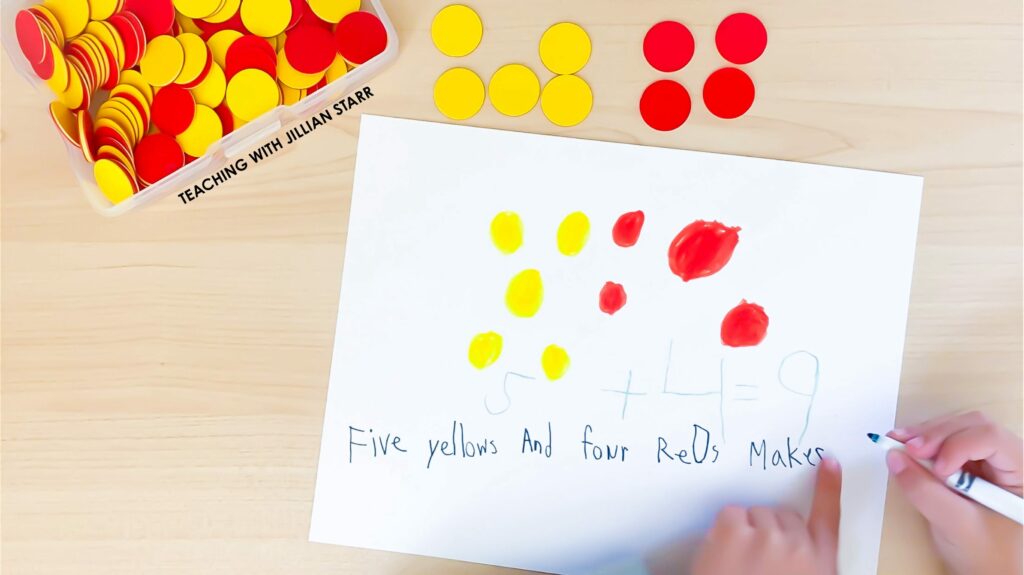
Now, our goal is to give those counters context. What could they be representing in real life? Perhaps “D’Andre wrote 4 thank you cards on Monday, and then wrote 5 more on Tuesday. He has written 9 thank you cards.” This shows a translation between the concrete counters and a contextual representation.

We could also be providing the context to their visual, their number sentence, or their verbal explanation. The point is that we are deepening student understanding by helping them connect one representation to a contextual one.

Connection Two Contextual Representations

In every post in this series, we have focused on connecting different representations AND how to support connections within the SAME representation. Here, a student could connect their previous context of D’Adnre’s thank you cards to additional contexts.

For example, “Liana read four books last week and this week she read five. She has read nine books in total.” By understanding that both situations give context to the same idea, the student has translated between two contextual representations.

What’s Up Next?

This series has gone on a dive deep into each of the representations discussed in Lesh’s Translation Model. Next, we are going to put it all together so we can make a big impact on your math teaching this year. Are you ready?

If you missed Part One about Concrete Representations, Part Two about Visual Representations, Part Three about Symbolic Representations, or Part Four about Verbal Representations, check them out so you have all of the info you need before we move on!