
Addition strategies are something we want all of our students to be using. But it’s essential that we really understand each of the strategies, as well as HOW and WHEN we want them to use different strategies. We’re going to start with a close look at the Compensation Strategy.
First, A Little Note About Addition Strategies
I’m going to share something that might make you feel a little uncomfortable, but stay with me on this journey! As our students begin to develop number sense and an understanding of number relationships, it can be really exciting to see them build a toolbox of strategies. However, it’s important that we allow these strategies to naturally develop.
There was a time that I wanted to explicitly teach each strategy and have my students practice it at length in hopes that they would use it… but the truth is that children are much more likely to use a strategy if they come to recognize its power on their own!
You might say, “But Jillian, what about students who DON’T come up with strategies independently? How do I get them to use addition strategies without explicitly teaching them?”
This is where Number Talks are a critical part of our math block! For our discussion, I will strategically choose an addition problem that lends itself to one or two particular strategies. When a student demonstrates the use of a particular strategy, we make sure to highlight it and why it’s such an efficient strategy for this problem. (Sometimes we even name the strategy after the student who shared it!)

An additional step can be to take a look back at a previous number talk problem and talk about whether or not this would be an efficient strategy for *this* problem. It’s a great opportunity for students to start understanding that we don’t develop strategies to use for all problems.
We don’t need students to apply each strategy to a single problem. Instead, we need to help students look at number relationships. The more we understand the relationships between numbers, the better we will be at selecting specific strategies for specific problems. Context matters!
What is the Compensation Strategy?
Compensation involves adding or subtracting from numbers to make calculations easier to work with. We typically use compensation to work with friendly numbers (sometimes called benchmark numbers), like multiples of five or ten.

Compensation is a two-step process. In the problem above, a student is working to solve 29+46. They notice that 29 is close to a multiple of ten, so the first step is to add one to 29 to create a new problem: 30+46. This problem is much easier to solve!
However, in the end, the student needs to remember that because they added one, they now need to take a second step and subtract one from their total. 76-1=75. This student has used the compensation strategy to solve their problem. They altered the number and then corrected the balance at the end to find their answer.
Note: This is different than a student who changes 29+46 to 30+45. That is the Transformation Strategy, which I talk more about it in this post!
Compensation can be defined as a method of adding or taking away numbers to make the calculation simple. Compensation in math often helps to reach a friendly number (such as a multiple of 10).
What does the Compensation Strategy look like in 1st Grade?
When I think about the Compensation Strategy with my previous first-grade classes, the first thing that comes to mind is the “Doubles Plus One” and “Doubles Plus Two” strategies. Can you see it?

When students see a problem like 6+7, they are taught to change it to 6+6 because many know their doubles facts. After they recall the sum of 6+6, they go back and add one at the end to compensate for the one they initially subtracted. Cool right?
It’s really powerful how observing the relationship between numbers like doubles in first grade can create a foundation for solving more difficult addition problems using the compensation strategy!
What does the Compensation Strategy look like in 2nd Grade?
Similar to the first example we looked at, students in second grade are often working with two-digit numbers. This can look like 27+9 or 27+59. In both cases, the compensation strategy would be an efficient strategy for students to use.
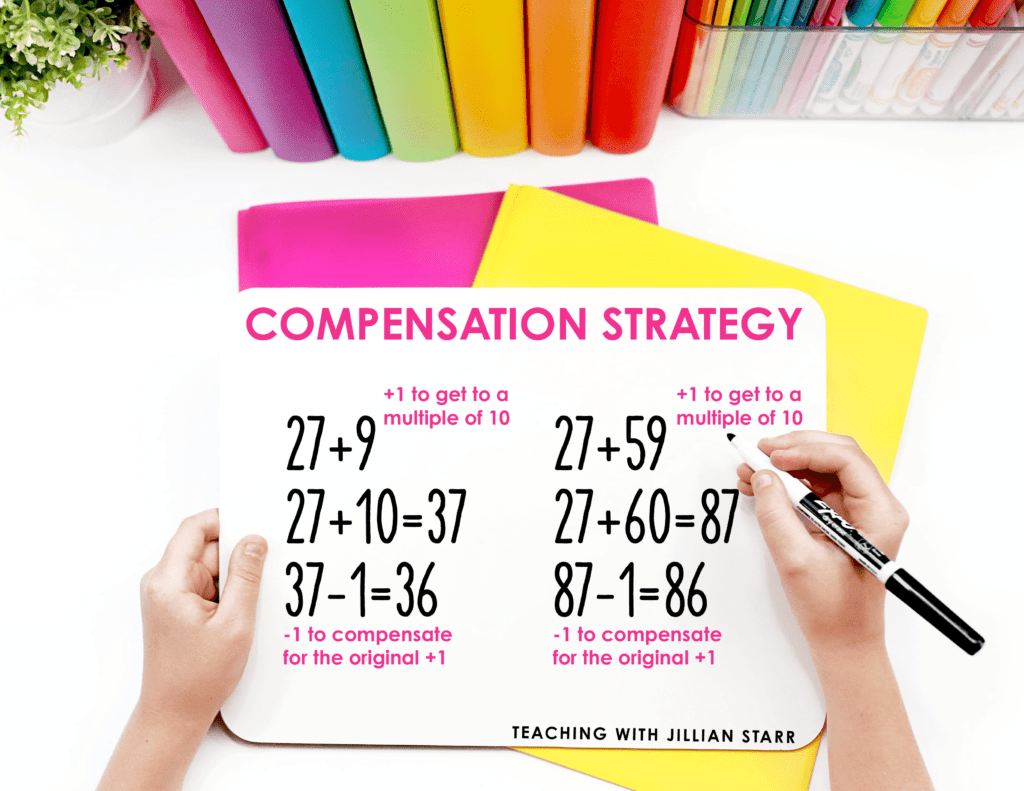
For 27+9, a student would likely add one to the nine to create a new problem: 27+10. Once they’ve solved for 37, they then have to compensate by subtracting one. 37-1=36.
When a student is working to solve 27+59, they can follow the same pattern of thinking. 59 is very close to a multiple of ten, so changing it to 60 will make it much easier to work with. 27+60=87, and then we finish the compensation strategy by subtracting one. 87-1=86.
What does the Compensation Strategy look like in 3rd Grade?
Third graders often move into three and four-digit addition, as well as multiplication and division.
Compensation Strategy with Addition
With three and four-digit addition, students may move beyond adding or subtracting single-digit numbers and compensate with larger numbers to find friendly numbers. For example, a student might encounter the problem: 3988+2435.

The student may choose to add 12 to create a new problem: 4000+2435. After solving for 6435, the student can compensate by subtracting 12 to get 6423.
Compensation Strategy with Multiplication
As students build number sense, they are more and more likely to start noticing relationships between numbers. The more they see those number relationships, the more likely they are to naturally develop strategies based on those relationships.
If a student finds success with the compensation strategy in addition, it may encourage them to attempt using compensation with other operations. For example, compensation comes up often with multiplication.

When students solve 7×9 by solving 7×10 and then subtracting 9, they are using the compensation strategy.

When they solve 38×3 by solving 40×3 and then subtracting 6, they are using the compensation strategy.
Closing Thoughts on Compensation
As I mentioned before, the compensation strategy is something we want students to discover organically. I don’t say this to shame anyone who is explicitly teaching addition strategies. I know that I was expected to explicitly teach them as part of my curriculum, and thought that I was supposed to have students learn to solve a single problem using a variety of different strategies.
However, what I learned over many years and multiple grade levels is that we actually DON’T want students to think that each strategy can be applied to any given problem. We want students to be thoughtful about which strategies make sense given the problem in front of them. We want them to critically consider what will be the most efficient way to solve the problem.
For example, the compensation strategy wouldn’t be the most efficient strategy for a problem like 34+55. Don’t worry! We’ll talk about more addition strategies, in upcoming posts.
For now, the big takeaway here is that we want to try and offer experiences for students to naturally discover these strategies and have opportunities to share their thinking with peers who may benefit from listening to new, more efficient strategies in an organic setting.
Exciting News!
First Grade Teachers, something big is coming! If you’re someone who loves digging into math concepts, or wants to become a better math teacher for your students, I’d love for you to join me. If you’d like to be the first to find out more, enter your info below!
Get on the Waitlist!
1st Grade Teachers! Something big is coming this June and I will be inviting a small group of teachers to get a first glimpse before it becomes available for everyone. If you’d like to be the first to find out more, just enter your info below!









Love your ideas and, for some, a reminder of that strategy!