
Oh, multiplication. It is a crucial part of mathematical development. Yet, supporting the construction of multiplication concepts can be surprisingly frustrating. That’s why I decided to stop focusing on rote memory. Instead, I decided to revamp my approach to incorporate hands-on ways to teach multiplication.
Memorization led to a stark imbalance in both students who could remember their facts and those who couldn’t. When it came time to apply their memorization to more complex problems, it was clear that they didn’t understand the underlying processes.
Knowing multiplication facts helps students efficiently solve mathematical equations as they advance. However, students hit a wall without solid number sense and a foundational understanding of how multiplication works.
Like all mathematical concepts, students build multiplication understanding through three stages: concrete → pictorial → abstract. Some students may naturally or more quickly see and develop an understanding of multiplication patterns. Others need a little more support. How do you scaffold this complex new understanding of math? Hands-on activities that show how multiplication works.
Today we will be talking about how to teach and foster strong foundational understandings of multiplication. To get you started, I’ve gathered my five favorite hands-on ways to teach multiplication.
Arrays with Square Tiles
There are a number of ways that arrays with square tiles support the conceptual construction of multiplication. I use square tile array building as a hands-on way to teach multiplication at the very beginning and as we move into thinking about factorization and multiples.
Arrays with tile squares are one of the first hands-on multiplication practices that I teach. I find it a particularly effective way to support early understanding of multiplication for two reasons:
- It is a hands-on method that uses one-to-one correspondence.
- Arrays with tile squares are a visual representation of the relationship between multiplication and repeated addition.
Bonus: Students naturally start to notice the commutative property (e.g. 3 x 4 = 4 x 3).

One-to-One Correspondence
This continues to be a crucial aspect of mathematical and number sense development for many students, even as you enter your multiplication unit.
I ask students to make X number of rows of X tiles. If one-to-one counting is still part of a student’s development, they can easily tap each individual tile to see how many there are in total. We do this in guided groups and then continue practice in centers. After building the array, I ask students to write a number sentence. In the beginning, I will have students practice making arrays by fact family (2s, 5s, 3s, etc.). However, there is a lot of flexibility! Students can use 10-sided dice to have more random number options as they become more familiar with the exercise.
The Relationship Between Repeated Addition and Multiplication
This relationship becomes evident as we build our arrays. To emphasize this relationship through hands-on teaching, I often have students leave a little space between rows. Students push them up as we “add” the rows together. When they record, they write both a multiplication number sentence and a repeated addition number sentence.
Square tile arrays are not just a hands-on way to teach multiplication in the early stages of understanding but can also be an excellent way to have students thinking about factors naturally. For example, how many different arrays do we notice make the number 24? Do you think we can come up with any others? Why do you think 24 has so many, while 23 only has two?

Multiplication Centers
Unifix Cube Trains
Readers of my previous math posts know that I love Unifix cubes. I’ve raved about their benefits for building number sentences, place value, addition, and subtraction. Now it is time for me to show you why you should be using Unifix cubes for multiplication.
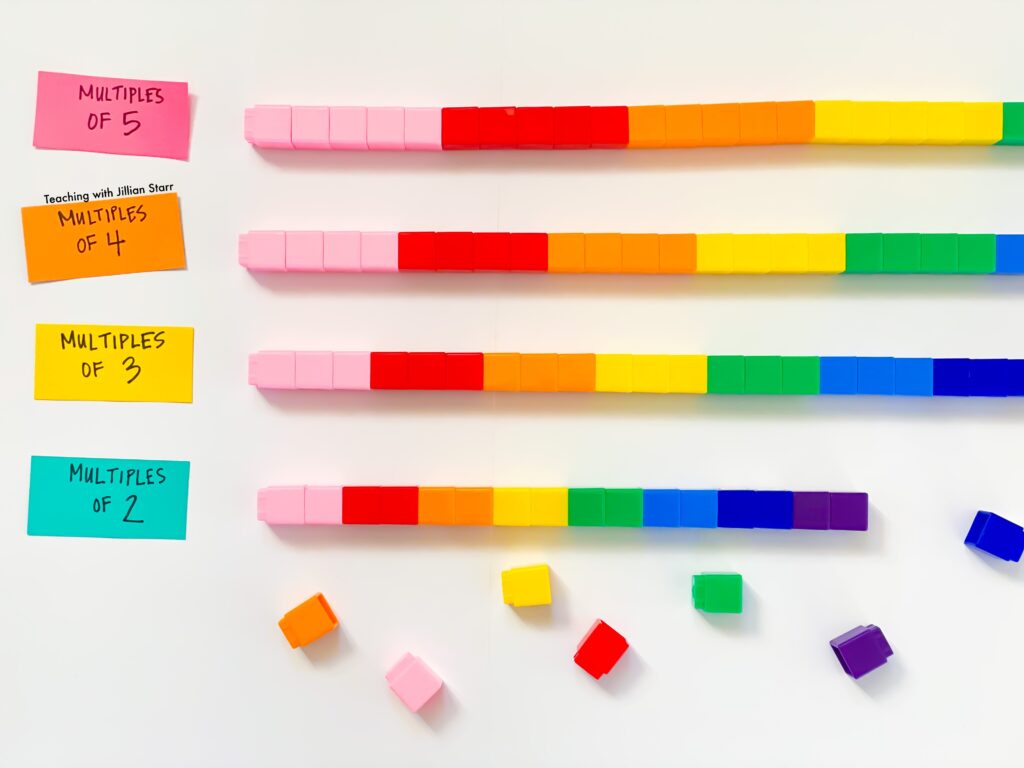

Unifix cubes visualize number lines. They connect vertically and horizontally in straight lines. Students can see mathematical operations in actions as they construct and deconstruct Unifix cube trains.
The best way to build an understanding of multiplication through Unifix cubes is by building number trains. I like to use them for practicing specific multiplication fact families. For example, if I am looking to understand the four times tables, I can create a train of cubes.
First, I ask students to build sets of 4 cubes (all different colors). Then, I ask students to add these sets together. Every group of four will be a different color to show the next group. This can help students see the group on a number line and understand skip counting by 4’s).
Array Towers and Cities
Let’s classify this hands-on way to teach multiplication as the most fun. I love building array towers and cities with my students. Students are motivated to keep practicing multiplication facts with the power of their imagination!
Array towers are paper rectangles that range in size (think: half of an 8 ½ x 11 paper and larger). On each rectangle are arrays of square windows. Students design their array tower and then add it to a series of other towers to form a city of arrays!


How does it work?
Prep: Cut out large rectangles of all different color paper. Cut a variety of different size black squares. The colorful rectangles are the buildings. The black squares represent windows.
Activity: Explain the paper representations (building and windows). Ask students to arrange arrays out of the black squares on the color paper, explaining that these are windows. Remind students that arrays are equal rows and columns. Once their array is complete, they will glue the windows onto the building. Underneath, ask students to write a number sentence to represent their array. Once everyone’s array tower is complete, design your skyline together!
Bonus: This makes an excellent, functional bulletin board! I often see my students linger around the bulletin board, admiring the buildings and counting the squares. It is pretty with a purpose!
Multiplication Legos
Get ready for some very excited students. Why? Because legos are a not-so-secret hands-on multiplication practice to have in your bucket.
After building a solid foundation in understanding arrays, I like to pull out our box of legos. Legos can build on the concrete understandings built with square tiles, Unifix cubes, and our array cities. They can take these concepts a step further.


First, Legos are natural arrays. Their connection points form arrays. A straightforward method of using legos is by looking at them. I love to dump a bucket of legos on a table (or floor) and work with students in identifying arrays. The arrays range from 1×2 to numbers over 20. Get ready for some serious math!
Second, Legos have tremendous flexibility, making them the perfect tool for open-ended activities with multiple access points. Students can:
- Build 3D arrays
- Build array cities
- Identify true arrays
These activities can be open-ended and have multiple access points. Some students will want to stick to just saying which array a single lego represents, while others may want to put legos together to create larger arrays. Talk about differentiation!



Multiplication Centers
Array Scavenger Hunts
Students can find math everywhere— at least, that is what I want them to grow to believe. And scavenger hunts for mathematical concepts are a simple way to get your students to start noticing math in the world around them. Multiplication is no exception.
I start with a discussion anytime I introduce looking for math in the “real world.” This helps to turn on students’ brains and get them thinking in mathematical ways. We start by focusing on the classroom–sometimes even just a wall. I ask students, Where do we see arrays in this part of our classroom?
This question sets them up to successfully notice arrays throughout the classroom independently. Typical arrays often include windows, cubbies, bins, rows of desks, and books on bookshelves (be careful to check the number of books in each row). You might have job charts, center rotation charts, and other wall resources that may also be in the form of an array.
Next, we explore the whole school. You will be surprised by the number of arrays students find. They notice arrays on bulletin boards, signage– everywhere!
Finally, I ask students: Where do you see arrays at home? This question could be posed as homework, or students could visualize their homes and name arrays that they can remember. Even if you don’t assign homework, get ready for students to come in with excited cheers of “I noticed that _________ was an array!” One of my students came in after a long personal scavenger hunt announcing that they even found an array in the freezer: the ice cube tray!
I hope these tried-and-true multiplication practices help– whether you are just getting started using hands-on ways to teach multiplication or you’re looking for something new. Have another hands-on way to teach multiplication? Please share!
You can find even more favorite multiplication activities HERE!







Thanks Jillian, I love all these ideas especially the array cities and the scavenger hunt. It’s great to have some new ways to approach multiplication. You’re right, ‘just learn your times tables’ isn’t going to cut it for a lot of students.
I am so excited about the 5 ways to teach multiplication I just read about. Thank you so much.