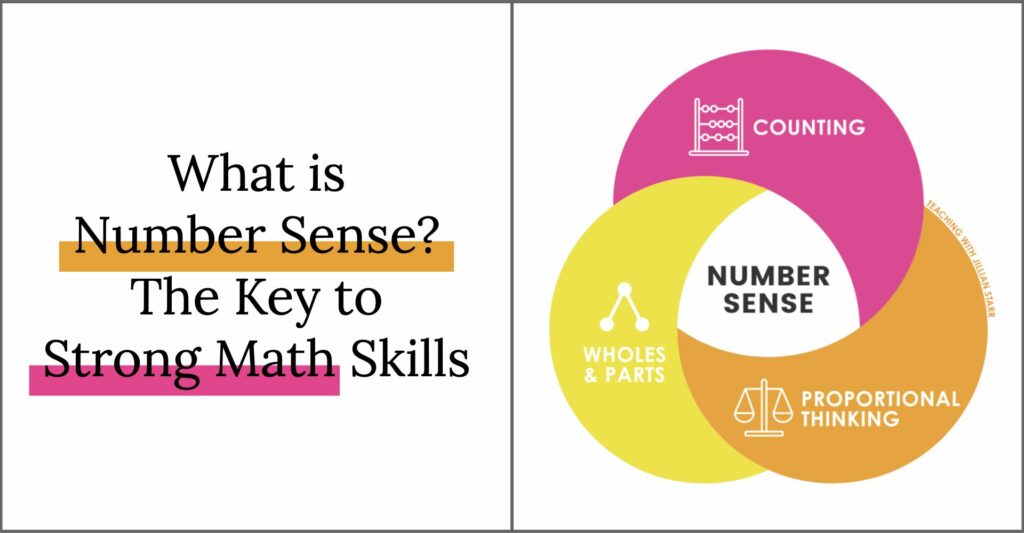
Have you ever heard the term “number sense” and wondered what it meant? In simple terms, number sense is an essential part of developing strong math skills. Number sense is an umbrella term to describe our understanding of numbers and our ability to think flexibly about them. Number sense is comprised of three main parts—counting, proportional thinking, and understanding part-part-whole.
As young children progress through the primary grades, they follow a progression of skills that are necessary to build strong number sense. Let’s define some of these key skills and explore how they build upon each other to develop strong number sense.
Number Sense and Counting
Counting is a large component within the umbrella of number sense that encompasses far more than the rote counting sequence. (Ask any pre-k or kindergarten teacher, and they’ll tell you!)
What is One-to-One Correspondence?
When a child demonstrates one-to-one correspondence, they are able to count one object for each number in the correct sequence.
A child with one-to-one correspondence has learned the number words in the counting sequence. They also understand that each object is counted only once, and that the order in which they’re counted doesn’t change the outcome.
As a student builds this area of number sense, they develop a strategy (or multiple strategies) to keep track of given a pile of objects while counting. It is important to note that different situations may require different strategies. For example, a student may pull one object close to them at a time while counting a pile of cubes, but they cannot use that same strategy when counting dots on a dot card.
What is Cardinality?
Cardinality is the idea that the final number used to count a group of objects tells how many there are. Essentially, cardinality combines the important skill of one-to-one correspondence with an understanding of quantity.
In the progression of number sense skills, students with an understanding of cardinality recognize that when they count six blocks, “six” is not the name of that last block, but the quantity of the entire pile of blocks. For students without a solid understanding, “six” holds no meaning for them. The purpose of counting is not clear.
What is Conservation of Number?
Conservation of number understanding that the number of objects remains the same, even when those objects are moved, spread out, rearranged, or some are hidden (e.g., 6 blocks in a pile is the same amount as 6 blocks spread out).
This can be a difficult concept within number sense for students to grasp, Often students see the spread-out blocks and automatically assume that there are more because they take up more space. Students need multiple experiences with counting the same amount in different arrangements to believe and internalize this concept.
Conservation of number will continue to develop as students build more advanced counting strategies, like counting by groups. As students’ number sense develops, they will begin to understand that you can count to the same number in multiple ways. For example, you can count fifty-three objects by ones, but it is far more efficient to first place the objects in groups of ten and then count by tens and leftovers.

Counting and Number Recognition Activities
What is Number Recognition?
Number recognition is the ability to identify and connect numbers verbally (number name), symbolically (written numeral), and visually (a representation of quantity). It is a comprehensive skill, far more than identifying numerals or writing numbers. It involves the connection between the understanding of quantity and how we express that quantity.
Number Sense and Proportional Thinking
With proportional thinking, we move on from “how many” to comparing quantities. When focused on counting, students are typically looking at numbers in isolation. As we move into proportional thinking, we are asking students to see the relationship between numbers.
What is Hierarchical Inclusion?
Hierarchical inclusion is the understanding that each number includes all of the numbers that come before it (e.g. the number five has the numbers four, three, two, and one nested within it). While hierarchical inclusion plays a huge part in counting and understanding quantity, it plays a major role in their understanding of number relationships.
When we begin asking students to change one number to another (e.g. “You have three blocks. How can you change that to five blocks?”) A student who doesn’t understand hierarchical inclusion will try to change three to five by adding five cubes, not realizing that the new quantity is eight. This student doesn’t understand that they don’t have to make a whole new pile to get to five if they already have three.
What is Comparing Numbers?
When we focus on comparing numbers, we are asking students to see the relationship between two quantities. Students may not have the language of “greater than” and “less than” to describe these relationships, but they are able to understand which is more and which is less. As their number sense builds, they will also develop an understanding of how many more and how many less.
Number Sense and Part-Part-Whole
As students build on their understanding of counting and proportional thinking, we want them to start considering the part-part-whole relationship.
What is Subitizing?
Subitizing is when we instantly recognize the number of objects without actually counting them. We can subitize numbers through five, and after that we are actually recognizing PARTS of numbers. For example, we cannot immediately subitize 7, but we can subitize a 5 and 2 within the 7, or the 3 and 4 within the 7, depending on how its organized and how your brain interprets the configuration.
Subitizing combined with the understanding of hierarchical inclusion provides a strong foundation for being able to see the relationships between parts and wholes.

Composing and Decomposing Math Centers
What is Composing Numbers?
To compose numbers is to put parts together to make a whole. We want students to see that we can combine parts to create larger amounts, and that larger amounts can be made up of smaller amounts. As students begin composing numbers, we can encourage the development of strategies:
- Counting On
- Commutative Property
- Doubles and doubles +1
- Related Facts
What is Decomposing Numbers?
Decomposing numbers is when we break apart numbers into smaller parts. When building strong number sense, we want students to think flexibly about numbers, and recognize how we can decompose numbers in different ways. For example, we want students to understand that six can be decomposed into one and five, two and four, or three and three.
The ability to internalize combinations through ten is an essential piece of foundational number sense. This flexibility with numbers will support students as they build more advanced mental math strategies.
Number sense is a critical piece in building strong math skills in elementary school which will carry through into higher levels of mathematics throughout life! It’s important to give students time to build these skills. Building number sense cannot be rushed. While we can guide them, our students must arrive at each of these concepts and skills on their own.
How Can We Build Number Sense in the Classroom?
There are a variety of experiences we can offer students to help them build number sense in the classroom. These can take place in whole group settings, targeted small group instruction, or at independent math centers.
Building Number Sense in the Whole Group
My favorite way to build number sense in the whole group is through Number Talks. Simply showing different representations of numbers on dot cards is an excellent way to ignite powerful discussions. While students won’t realize it, they’ll be tackling important concepts we previously discussed like subitiizing, conservation of number, and composing parts.
Building Number Sense in Small Groups
I talk a lot about my love for small-group instruction around here. Small groups are the perfect place to:
- Provide targeted instruction around specific number sense skills
- Correct misconceptions
- Introduce new experiences that students can carry over into their independent work
- Quickly assess student understanding to guide instruction
Building Number Sense at Centers
Providing meaningful experiences at centers is going to be key! As I said before, building strong number sense takes time. Students need lots of exposure to concepts and time to work through them in order to believe and internalize those concepts.
If we want our students to truly build these skills and not just memorize sequences and facts, we need to build in time for it to happen. If you’re looking for some favorite center ideas, I’ve shared several in these posts:
If you are looking for help creating any of these activities, you can find some of my favorites below:
I hope this post has been helpful in breaking down some of the skills needed to build strong number sense. The more we can invest in our students’ ability to understand and think flexibly about numbers, the stronger their number sense will become!











Thank you for an excellent post that breaks down number sense into an easy progression of the knowledge students need in order to interact with ‘math’
Hi Sarah!
I’m so, so happy to hear that this post was helpful! Thank you for taking the time to leave these kind words.
Warmly,
Jillian
Fantastic, makes these concepts easy to explain to students.
Thank you!
I hope you all feel better soon!
I teach on the Spanish side of a dual program & it’s been ages since I have taught math. This is such a great explanation and refresher! Thank you so much!