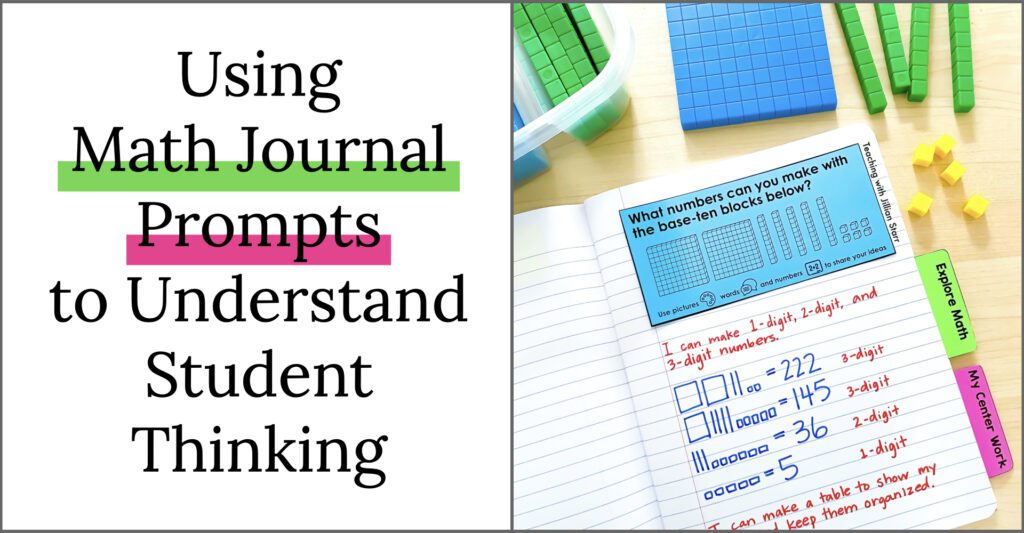
What if I told you there was a strategy that would revolutionize your math assessments? It involves no extra time and NO testing. Plus, it unlocks the answers to your biggest questions around math thinking.
Let me introduce you to daily student math journal prompts.
Daily math journal prompts are not only a staple in my guided math centers but my go-to tool for informal assessments. I noticed that student math journals acted as an engaging way to practice concepts while giving me a daily peek inside math thinking. Not to mention, the journals gathered data on computation skills and accuracy as well.
Today, I want to share three ways in which your students’ math journals give you all the information you need to know about your students’ math thinking.
Bonus: I will provide you with some questions you can ask to help you get the most information from student math journals.
Are My Students Thinking Flexibly?
When looking at student work, there are many aspects to consider. How accurate is this student? Do they understand the procedures for this computation strategy? Do they know what is being asked of them? And often, we ask: did they get it right?
Often the “right or wrong” approach to analyzing student work misses the mark on a crucial aspect of mathematical thinking development. A significant part of math fluency is flexible thinking. The ability to see a problem, understand the problem, and solve the problem in multiple ways.
That’s where flexible thinking comes in. We want to train our students to understand patterns and problems so well that they can see a problem existing in multiple ways. Sometimes with multiple answers. And then be able to approach the problem in various ways to find a solution. And to be able to do this over and over again!
That’s where your student math journal and open-ended prompts become crucial. Open-ended prompts are the most significant benefit of using student math journals. Students choose their own set of numbers, and there are unlimited ways to solve the problem. They require flexible thinking.
Problems that can be solved in unlimited ways are the best way to assess how flexible math thinking is. Why? Because you are not looking for a specific answer.
Instead, you are asking students to interpret a problem. You want them to get creative and reach into their mental (or physical!) toolbox to find a solution. And then another…and another…and another… Students are never done! Until the time is up, they are encouraged to continue looking at a prompt to find new ways of answering it.
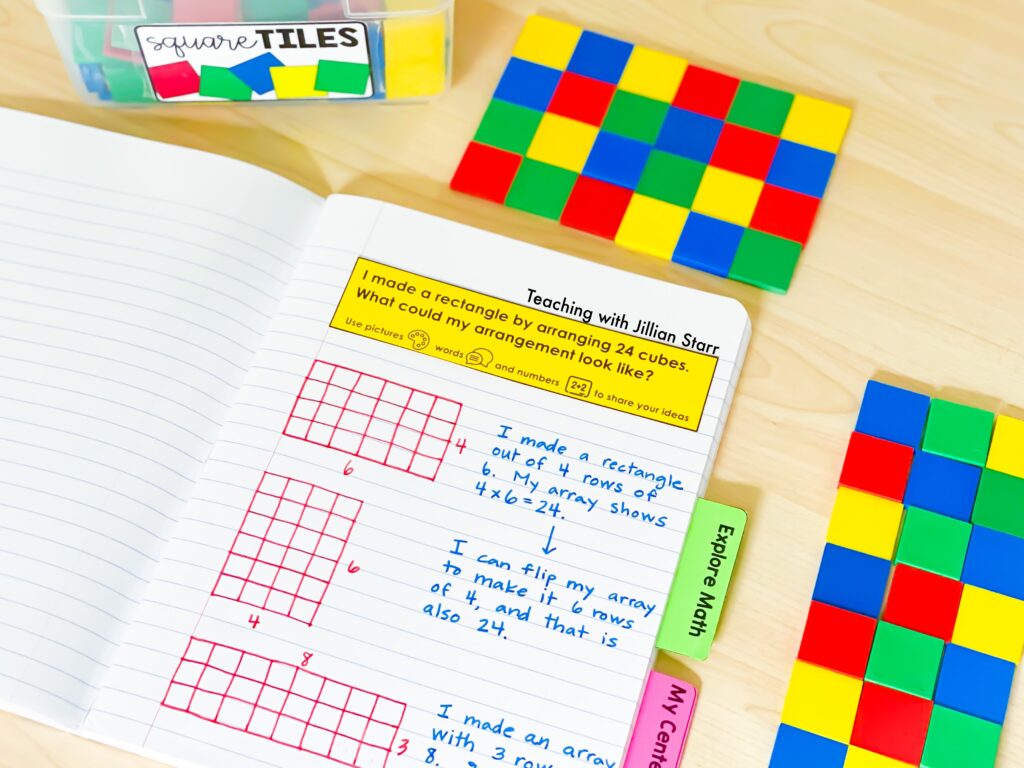
This shows us:
- The ways a student can interpret a problem.
- The patterns students are identifying within the problem.
- The ability to see multiple ways to approach a problem or see patterns within a real-life scenario.
- The likelihood of a student accurately applying the same strategies to another problem following a similar pattern.
It can be hard to know what to look for. Here’s a couple guiding questions to start analyzing student math journals for flexibility:
- What approach is the student using to solve the problem?
- Can the student use more than one approach to solve the problem?
- Is the student using multiple strategies, numbers, or coins (if applicable) to prove or show thinking?

Math Journal Prompts (Grade 2)
Where are the GAPS in my students’ understanding?
Every kind of student math journal prompt will provide you with a unique type of information. Closed and semi-closed math prompts provide accuracy-based information and work well as test prep. I, however, choose open-ended prompts.
One big worry about including (or relying on) open-ended prompts is that students won’t show their misconceptions. This couldn’t be further from the truth.
Before incorporating math journals and open-ended prompts, I was only scratching the surface of understanding how my students were thinking about and comprehending our work in math.
First of all, once a student has learned their math journal routine, they will begin putting more (and more and more!) thinking down on paper. I have noticed that the naturally differentiated aspect of open-ended math journals encourages students to persevere through a challenge. Every attempt to solve a problem in a new way or with new numbers provides me with more data on how a student is thinking.

When students show us their thinking, they show us what they can and cannot do. This goes beyond the ability to compute. The strategies they choose to use in response to a prompt show us the methods they feel comfortable using. After that, their work shows us whether or not they can use these strategies effectively. After all, they have picked numbers and methods that feel comfortable. If there is still a disconnect, where is the missing link? Finally, the strategies and numbers students use tell us how they are interpreting a math problem. Their choices then show whether or not they truly understand how to use numbers and operations to solve it (or a new method that worked).
Some questions for analyzing student math journals for gaps in understanding and misconceptions:
- What is the question this student is attempting to answer?
- Does this student include all components of the open-ended prompt? Or is something missing or changed?
- Are they using math strategies with accuracy? Could their strategies work with other numbers? If not, where is the trouble spot?

Math Journal Prompts (Grade 3)
How willing are my students to CHALLENGE themselves?
Open-ended math journal prompts are kind of like a “choose your own adventure.” Instead, it is: Choose your own challenge!
Prepare yourself for two things. You won’t regularly hear those common phrases such as “I’m done,” “I can’t do this,” or “This is too easy”. Since students can choose their own challenge, they decide what kind of challenge they are ready for.
Responses to the same prompt can look very different simply because students adjust to their own comfort level. Let’s look at an open-ended problem for third grade as an example:
Together, Anna and Nicole have 900 stickers in their collections. Use pictures, words, and numbers to share your ideas.
A less advanced or less confident math thinker might choose simpler numbers and equations. For example, they might write about how Anna has 400 stickers, and Nicole has 500 stickers. 400 + 500 = 900.
A more advanced or confident math thinker might choose the challenge of more complex numbers or ways to create or manipulate the number 900. For example, they might write about how Anna has 431 stickers, and Nicole has 469 stickers. 431 + 469 = 900.

Since open-ended prompts encourage students to attempt more than one response, you can see how students grow within their thinking. For some students, you will see that their first response is pretty simple. It acts almost like a warm-up. Then, they try strategies or numbers that are a little more… adventurous. For other students, their math journal work might show an instant need for a challenge. You might see that they interpret the prompt as a complex problem (for better or worse) because they want the toughest challenge of all. And others… well, they might stick to numbers that leave less room for error.
Keep in mind:
Reasons for choosing less challenging numbers aren’t always a sign of ability. Some students will use a limited range because they are worried about challenging themselves. Sometimes students interpret questions in more straightforward ways.
Some questions for analyzing student math journals when considering challenge:
- What numbers or coins is this student choosing to use?
- Is this student using pictorial, number line-based, or abstract computational strategies to respond to this prompt?
- Does this student pick the same strategies over time, or do they try new ways of solving related prompts from day to day?
- Is this student practicing strategies we have reviewed in a whole group or small group? Or, is this student relying on early methods that might feel safer?
I hope this post has given you some food for thought on making your student math journals an even more powerful tool in your classroom. This fall, take some time to get to know your students through their math journals. And if you spot additional ways to discover how students think about math, please share with us!











I thoroughly enjoyed reading your post on not using keywords. I am already on that hill because I saw the confusion using keywords presented when I started using them. So, I stopped using them as guide to solving the problems. Instead, we have been organizing our understanding through drawings and acting the problem out for 5 minutes each day. I have utilized wordless problems but not as much as I should. I intend to buy your Math Prompts, to work with me students. I am excited about it as a matter of fact.