Mathematical Representations Series Part 3: Symbolic Representation

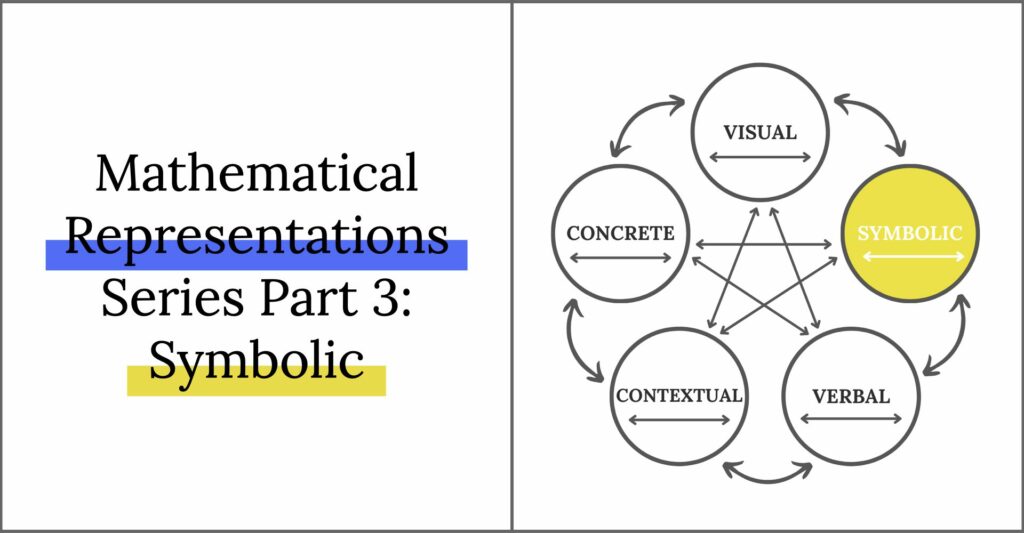
Welcome back to our deep dive into mathematical representations! Today, we are taking a look at symbolic representations and how we can translate between symbolic, concrete, and visual representations. First, let’s do a two-sentence recap of this series so far:

We have already focused on concrete representations and the immense value of manipulatives, as well as the range of visual representations we want to encourage with our students. We are centering our conversation around Lesh’s Translation Model, which encompasses the range of ways we represent our thinking, and stresses the importance of making connections between representations.

Symbolic Representation

Now, that we’re all caught up, let’s take a peek at Symbolic Representations. You may have heard it called Abstract Representation, especially if you’re familiar with the Concrete-Pictorial-Abstract model (also called CRA model).

So what are symbolic representations? Well, symbolic representation is when mathematical symbols (like numerals and operation signs) are used to show a mathematical concept. They are using symbolic, mathematical language to express their understanding of a math concept.

Examples

Let’s go back to the example from concrete representation. In that example, the student used 5 yellow counters, and 4 red counters to concretely show their understanding of 5 + 4 = 9.

Then in their visual representation, they were able to create a sketch of their concrete representation. This was our first example of translating between two different representations (connecting visual to concrete).

Now, we can support students even further by helping them represent their understanding with symbols. Here the student counts the collection of five counters and writes the numeral “5” below it. They do the same for the four counters.

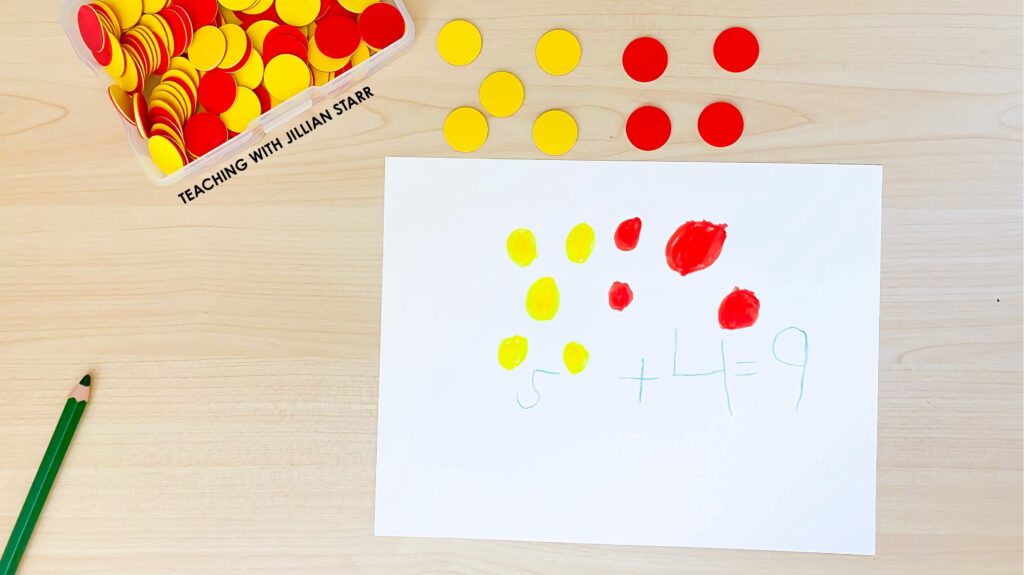
Finally, they count all of the counters together and write down “9”. We can help them with the operations symbols if needed, showing that “+” means that we are putting the 5 and the 4 together and that the “=” means “4+5” has the same value as “9”.

When we guide students to using numerals and operations, they are connecting their manipulatives, their sketches, AND the symbolic representation. This is such a powerful move to help deepen student understanding!

Connecting Two Symbolic Representations

It’s a fairly straightforward representation, but how do abstract representations connect to themselves in Lesh’s translation model? 

Well, this student actually wanted to write 4 + 4 +1 because that is how they solved the problem. They knew 4 + 4 = 8, so 4 + 5 = 9 because it’s 4 + 4 + 1 more. Understanding that both expressions can describe the same concept is just one example of how to translate within the symbolic representation.

What’s Up Next?

This series is going to dive deep into each of the representations discussed in Lesh’s Translation Model, and then we are going to put it all together so we can make a big impact on your math teaching this year.

If you missed Part One about Concrete Representations or Part Two about Visual Representations, check them out so you have all of the info you need before we move on!