
Unitizing- What is it? Why is it such a big jump for students? What role does it play in place value? What can we do to support students in developing this concept? These are just some of the questions we will answer in this article to help you understand the critical role unitizing plays in how students build an understanding of place value. Are you ready to dig in?
What is Unitizing?
Unitizing is the concept that a group of items can simultaneously be described as a number of smaller items and as one group. In simplest terms, it is the understanding that we can count groups as single units. An example of this is when we begin working with groups of ten. “Ten” is both a collection of ten smaller units, as well as a single unit with a value of ten.
Why is Unitizing Such a Big Jump for Children?
Let’s consider what children have been working on in pre-k, kindergarten, and the beginning of first grade. They have been building a foundational understanding of:
- 1:1 Correspondence
- Cardinality
- Conservation of Number
And more. When we start counting groups, especially as we approach teaching place value, we need to go back to these skills and almost define them for students.
For example, children have just learned to count one object for each number in the counting sequence (1:1 correspondence). They have just learned that “one” means a single object, and “two” means two separate objects.
As we introduce counting groups concerning place value, we are asking students to begin counting groups as single units. Now that “one” which used to mean a single object can now mean ONE group of multiple objects, for example, “one group of ten.” (Later that will expand into “one group of one hundred,” and “one group of one thousand.”)

This can be a huge jump for students. We are asking them to significantly shift their thinking about what counting means. We are asking them to now hold two truths at the same time: A ten is BOTH a single unit, AND a collection of ten smaller units.
What Role Does Unitizing Play in Place Value?
Unitizing is the foundation for place value. Place Value is based on the premise of a digit having different values depending on its position. The placement of the digit describes how many groups of a given value. For example, the 9 in 397 says that its value is 9 groups of ten (or 90). The 3 in 397 says that its value is 3 groups of 100 (or 300).
In order to conceptually understand each place’s value, we have to understand that each place is ten times greater than the place to the right. In the early grades, this looks like understanding that 10 ones equals 1 group of ten, and 10 groups of ten equals 1 hundred. If children are not yet able to understand that ten is both ten ones and one ten, they will struggle to develop future place value concepts. This has to happen first!
Standards that Include Unitizing
Now that you have a better understanding of unitizing, it probably won’t surprise you to learn that several standards involve unitizing. Let’s look at some examples from first grade:
CCSS.MATH.CONTENT.1.NBT.B.2 (A, B & C)
Understand that the two digits of a two-digit number represent amounts of tens and ones. Understand the following as special cases:
- 10 can be thought of as a bundle of ten ones — called a “ten.”
- The numbers from 11 to 19 are composed of a ten and one, two, three, four, five, six, seven, eight, or nine ones.
- The numbers 10, 20, 30, 40, 50, 60, 70, 80, 90 refer to one, two, three, four, five, six, seven, eight, or nine tens (and 0 ones).
CCSS.MATH.CONTENT.1.NBT.B.3
Compare two two-digit numbers based on meanings of the tens and ones digits, recording the results of comparisons with the symbols >, =, and <.
CCSS.MATH.CONTENT.1.NBT.C.4
Add within 100, including adding a two-digit number and a one-digit number, and adding a two-digit number and a multiple of 10, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method and explain the reasoning used. Understand that in adding two-digit numbers, one adds tens and tens, ones and ones; and sometimes it is necessary to compose a ten.
Do you see how unitizing is embedded in each of these standards? We see the same patterns when we look at second grade with skip counting and the conceptual understanding of one “hundred” and one “thousand.”
How Can We Help Students with Unitizing?
The key to unitizing is giving students lots of experiences navigating the idea of groups as single units. One way to do this is to have students sort objects into groups organically.
Unitizing with Counting Collections
We discuss Counting Collections across a variety of math strands. If you would like some background information about counting collections and how to get started, you can refer to these posts for more information:
- Counting Objects- Using Collections to Create Hands-On Experiences
- Counting Activities to Boost Deep Conversations
- 3 Meaningful Ways to Introduce Place Value
The purpose of Counting Collections with respect to place value is to give children to begin naturally organizing the objects in their collection into groups. This can initially be groups of any size, since we truly just want them to see groups as single units and also as collections of smaller units.

Through discussion and thoughtful questioning, we want students to make connections between counting the number of groups and counting their total number of objects.
Eventually, whether through their own practice or observing the work of their peers, students will begin to see the efficiency of counting by groups, especially groups of ten. With more and more experiences physically organizing their collections into groups, counting their groups, and finding totals, students will begin to internalize the concept of unitizing.
Unitizing through Number Talks
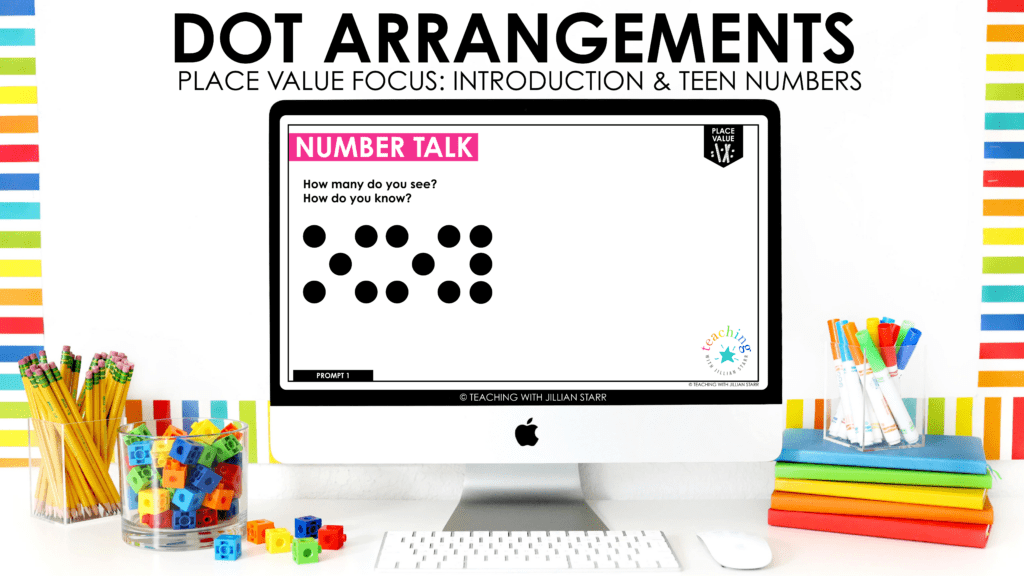
We have previously discussed how to introduce place value through number talks. Within our FREE set of Number Talk slides, you will find that the first ten prompts are completely visual. These slides are intended to help students begin seeing objects arranged in groups of ten and to encourage them to unitize.

FREE Number Talks
First grade teachers, access 20 FREE Number Talk Prompts to enhance your place value unit and get your students engaged in conversation.
These prompts are powerful reminders of different ways we can build the concept of ten throughout our school day! Utilizing visuals that can reinforce units of ten in the classroom environment can be a powerful tool (e.g. ten frames, rekenreks, fingers, bundles of ten…etc.). You can find several ideas in this post about adding ten-frames to your classroom routines!
Unitizing with the Hundred Chart
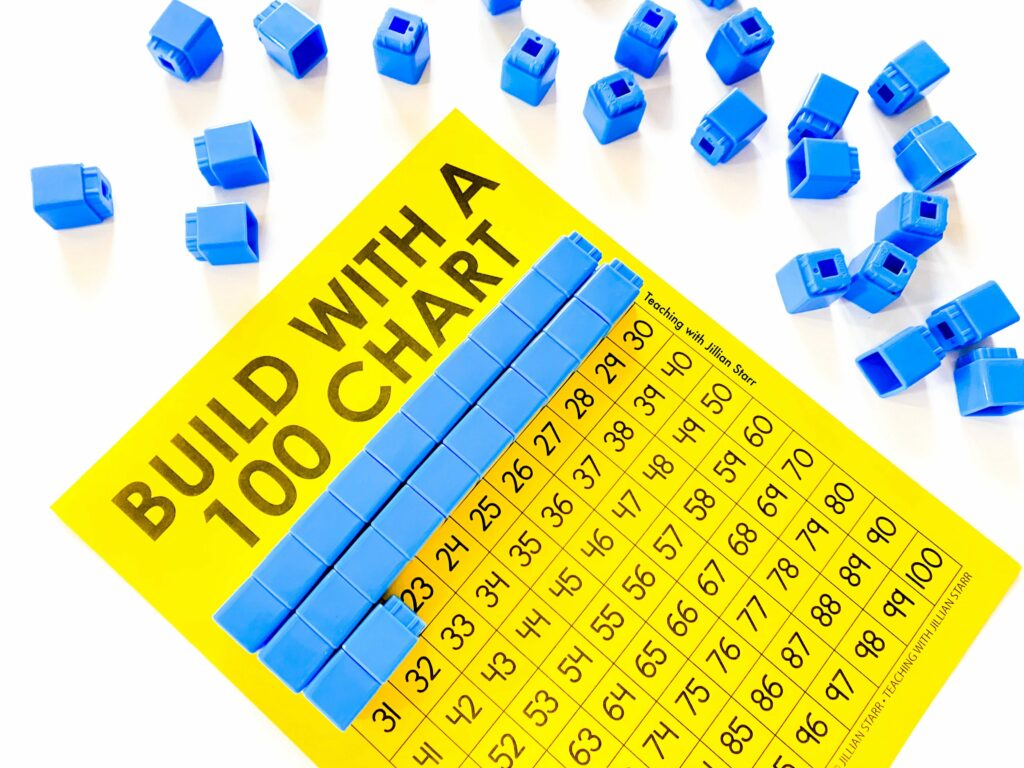

The hundred chart can be a very useful tool when teaching place value. If you’ve never seen how I like to introduce the hundred chart with Unifix Cubes, that will provide a great starting place.
A wonderful follow-up activity is to have students build groups of ten on their own hundred chart. I have made a hundred chart template (Click here to download for free) that easily fits Unifix Cubes and allows students to really see and experience how ten ones make a ten.


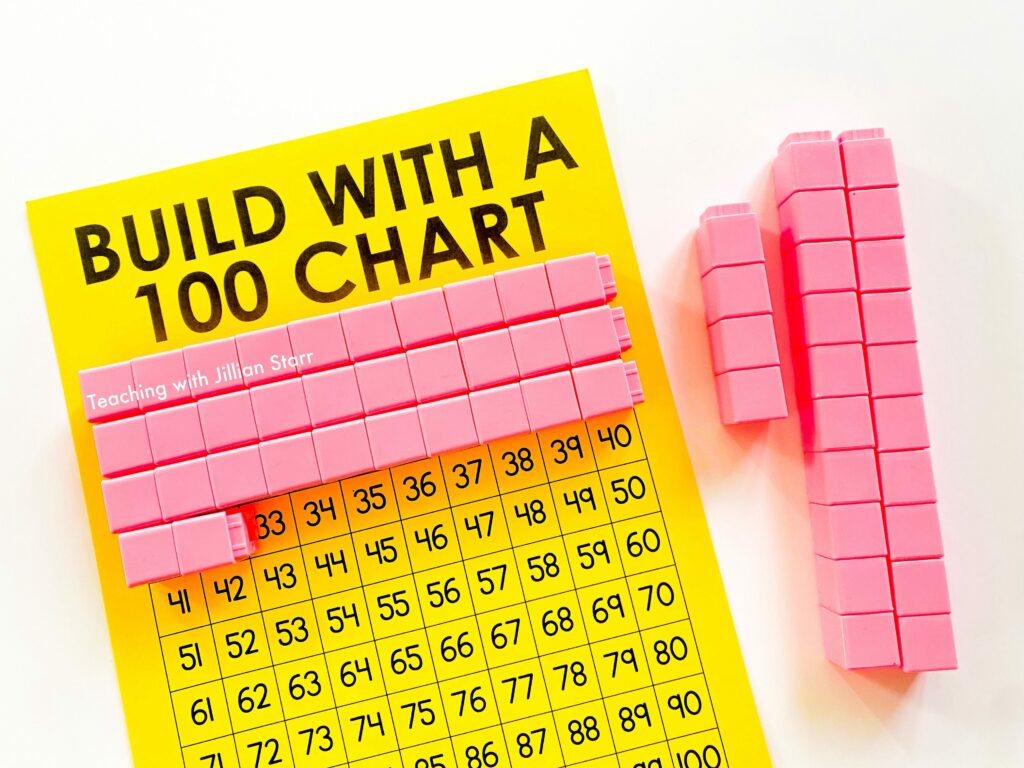
This can also be a great hands-on tool to start composing and decomposing two-digit numbers. For example, if I have 25 and I want to add 36, we can start by building each addend with Unifix Cubes. Visually we see the two addends in their different colors. Then we can compose these numbers on the hundred chart like in the image below and reinforce those groupings and regroupings of ten.


We can do the same for subtraction. We can build the minuend with our Unifix cubes on the hundred chart. For example, if we have the equation 56-24, we first build 56.


Then we can remove the amount of cubes in the subtrahend (24) and see our difference (32). Again, this reinforces the groups of tens and leftover ones.
I created these charts to perfectly fit Unifix cubes for this activity. If you’d like to give them a try in your classroom, here is the link to them on Google Drive (they’re free!).

When we help students understand and internalize the concept of unitizing, we essentially unlock the entire world of place value for them! It’s quite powerful when you stop and think about it!
I hope this deep dive into unitizing was helpful and has given you some ways to support the students in your classroom who may be struggling to internalize this concept. Did you have an ah-ha or big takeaway? I’d love to hear from you!









I’m interested in intervention ideas.