
Missing addends can be really challenging for young mathematicians. They need to understand and be able to do so many things in order to accurately solve these problems: the meaning of the equal sign, the meaning of addition, the connection of addition to subtraction, and how to count on just to name a few.
Today I’m going to talk about four things that help my students understand and accurately solve for missing addends. These strategies help students not only solve the problems but (even more importantly!) support their conceptual understanding.
The Equal Sign and Missing Addends
Before students can begin to solve for missing addends, students need to truly understand the meaning of the equal sign. Why? Because otherwise, students will get creative with reading equations like 2 + _ = 5. I’ve had students read equations like that as 2 + 5 = _ more times than I can count! They see the plus sign and the equal sign and assume the blank just means, “I should add the numbers and put the answer in the blank.” When students do not have a solid grasp of the equal sign, it makes understanding what these problems are asking much more difficult.
How to Bring the Equal Sign to Life
My favorite way to teach the meaning of the equal sign is with a simple balance scale. I write an equal sign on a sticky note and stick it in between the two sides. Now my simple scale has become the perfect visual tool for understanding the equal sign and solving missing addends!
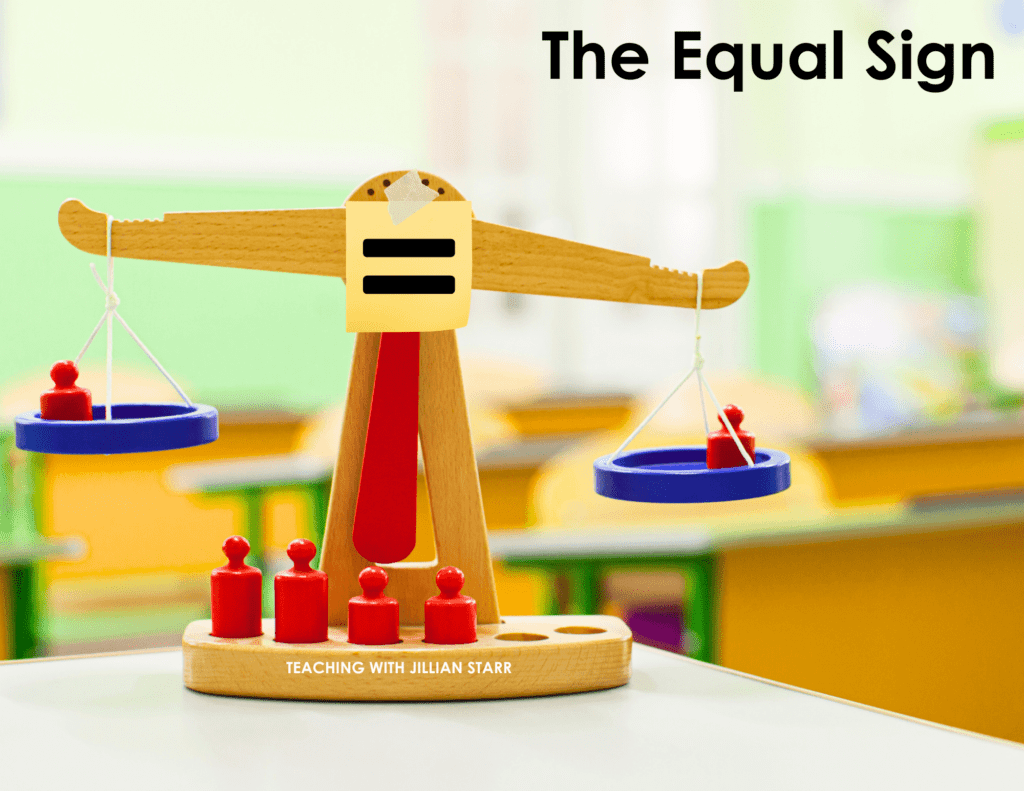
Here are the ways that I use the scale with students:
Introduction
The first time we use the scale, I present students with a problem like 4 + 2 = _. I ask them to use two different color cubes to show 4 + 2 and a third color to represent the sum on the other side of the scale. I let students know that if they are equal, the scale will be straight across like the equal signs.
True and False
We do a true and false activity. I show students equations and students need to determine if they are true or false using the scale to model their thinking. For example, I may show them 3 = 3. Students would then put 3 cubes on the left and 3 cubes on the right. This very simple equation can be very challenging for students who do not fully understand the equal sign. These students will likely put all 6 cubes on one side of the scale reading the equal sign as a plus and say the equation is false because it then does not balance.
When practicing with equations like 3 = 3, I will begin to narrate by saying something like “This equation is true because 3 (point to one side of the scale) is the same as (point to the equal sign) 3 (point to the other side). I can tell because it’s balanced.” This language begins to reinforce that equals means “the same as” instead of “the answer.” This true/false activity can be done with lots of other equations as well, such as 3 + _ = 7, 2 + 5 = 8, 4 = 2 + 1, etc.
Placement of the Equal Sign
Another common misconception is that the equal sign cannot come at the beginning of an equation. So, with my handy scale…we break that misconception! I ask students to use the scale to solve equations like 5 = 2 + _. Students put 5 cubes on the left side of the scale. On the right side, they use one color to place 2 cubes and then another color to represent the missing addend. A common error students make when they do not yet understand the equal sign is to place the 5 and the 2 cubes on the same side and then place 7 cubes on the opposite side. They will then write in 7 as the missing addend.
Working with Larger Numbers
Sometimes balancing the scale can get tricky, especially with larger numbers. It will just tip over from the weight of the cubes on one side. In this case, my easy fix is to hold the scale level and let go when the student is done modeling the problem. Then they will see quickly whether the scale moved and became unbalanced (i.e. unequal) or if it remained balanced (i.e. equal).
I repeat these activities constantly with different equations until students truly grasp that the equal sign means “is the same as.” This understanding greatly benefits their work with missing addends and with even more complex equations as they grow as mathematicians.
Using Context to Solve for Missing Addends
Context can turn a problem like 4 + _ = 9 from very confusing to crystal clear. How do we give students the context they need? Word problems! Word problems provide the real-life context that young students need to truly understand the math they are learning.
When using context, I provide students with various real-life situations that can be solved with missing addends (or subtraction!) equations. Let’s take this example:
Veronika had 4 crayons. Her friend gave her some more. She now has 9 crayons. How many crayons did her friend give her?
With the context provided, students can visualize the situation and can more easily determine how they could act it out or represent it on paper. Some students may actually get crayons to act it out. Others may use the balance scale and cubes. Even others may use a tens frame with counters, draw pictures, or just use their fingers. Regardless, the context of the story provided them with the information they needed to understand what the equation 4 + _ = 9 means.
At the same time, I provide students with multiple opportunities to “be the teacher” and come up with their own missing addends word problems. While I don’t expect kindergarteners and first graders to physically write their problems, I do expect them to think of their own additional situations and I record the problems for them. Not only do students love having their classmates solve their missing addends problems, but the challenge of coming up with their own deepens their understanding of the connection of the meaning of these types of equations.
Make Missing Addends Come Alive with Modeling
There is definitely some overlap between modeling and the balance scale but it’s important to emphasize a couple of other ways that students can use modeling to solve for missing addends.
In addition to using cubes with a balance scale, students can use cubes or counters to model missing addends on their own or with a tens frame. Either way, I emphasize the importance of using different colors to model the two addends. Without using two colors, students may show one addend, add on some more to find the missing addend, but then not be able to tell how many they added on because all of the cubes or counters are the same color.
Let’s go back to our problem from earlier: Veronika had 4 crayons. Her friend gave her some more. She now has 9 crayons. How many crayons did her friend give her? The images below show how students may model their thinking using cubes and counters.
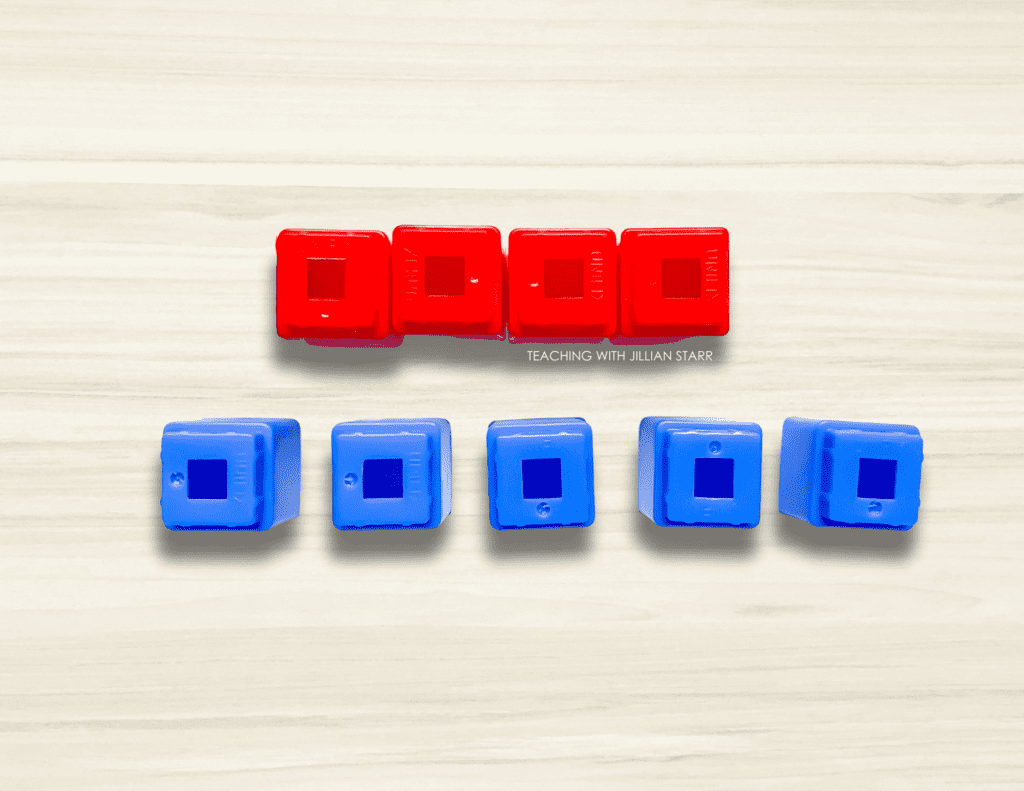
Students should also practice using drawings to represent their thinking. Similar to using different color cubes and counters, I emphasize to students that they should somehow make the two addends clear in their drawing so that they can solve for missing addends.

Below is an example of how a student might model their thinking about our crayon problem using drawings:

Using Equations to Solve for Missing Addends
Finally, when students are solving word problems, I ask students to write equations to match. With missing addends, writing equations can be tricky for students. Slowly writing the equation to match what is happening in the story they are solving can be helpful in accurately matching the equation to the word problem.
Using a line or box for missing addends can also help students to understand that the answer to the question goes in that spot. Without the line or box, I have found that students will write the equations as a two-addend addition equation and identify the sum as their answer.
I refer to the answer to the question as the “mystery number.” I tell students that there is a mystery number that we need to figure out using the clues the math story gave us. Calling it a mystery number has helped me to prompt students when they select the wrong number as their answer.
Students who do not fully understand the meaning of the equal sign will always say the answer to the question is the sum, regardless of the actual story. When I notice a student doing this, I’ll ask them if the number they say is their answer was the mystery number or if it was in the story. The prompt, “What is the mystery number?” will often get students to think again about what the question was asking rather than assuming the sum is the answer.
Writing missing addends equations themselves greatly supports students in understanding and solving missing addends without context. It bridges these types of problems and supports students in deepening their understanding of a deceptively simple equation like _+7=11.
So there you have it! Four strategies that can take the fright out of teaching missing addends. They all work together to help students to not only accurately solve problems, but more importantly, to help them to truly understand what a missing addend problem is asking them to do.
Let me know if you give these ideas a try. I hope your students begin to master missing addends so that that phrase stops making you cringe.
Looking for even more information about addition? Check out these posts:










Leave a Comment